Directions for question (1 to 5): All the first-year students
in the computer science (CS) department in a university
take both the courses (i) AI and (ii) ML. Students from
other departments (non-CS students) can also take one
of these two courses, but not both. Students who fail in
a course get an F grade; others pass and are awarded
A or B or C grades depending on their performance. The
following are some additional facts about the number of
students who took these two courses this year and the
grades they obtained.
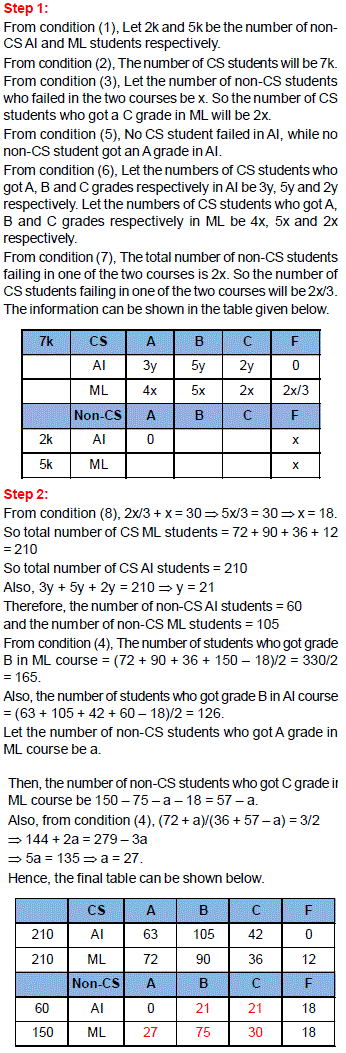
1. The numbers of non-CS students who took AI and
ML were in the ratio 2 : 5.
2. The number of non-CS students who took either AI
or ML was equal to the number of CS students.
3. The numbers of non-CS students who failed in the
two courses were the same and their total is equal
to the number of CS students who got a C grade in
ML.
4. In both the courses, 50% of the students who passed
got a B grade. But, while the numbers of students
who got A and C grades were the same for AI, they
were in the ratio 3 : 2 for ML.
5. No CS student failed in AI, while no non-CS student
got an A grade in AI.
6. The numbers of CS students who got A, B and C
grades respectively in AI were in the ratio 3 : 5 : 2,
while in ML the ratio was 4 : 5 : 2.
7. The ratio of the total number of non-CS students
failing in one of the two courses to the number of CS
students failing in one of the two courses was 3 : 1.
8. 30 students failed in ML.
Correct Answer
1
Explanation
Number of students took AI = 210 + 60 = 270.
Correct Answer
12
Explanation

Number of CS students failed in ML is 12.
Correct Answer
27
Explanation

Number of non-CS students got A grade in ML
is 27.
Correct Answer
1
Explanation

Number of students got A grade in AI is 63 + 0
= 63.
Correct Answer
4
Explanation

Number of non-CS students got B grade in ML
is 75.
Directions for questions (6 to 10): Pulak, Qasim, Ritesh,
and Suresh participated in a tournament comprising of
eight rounds. In each round, they formed two pairs, with
each of them being in exactly one pair. The only
restriction in the pairing was that the pairs would change
in successive rounds. For example, if Pulak formed a
pair with Qasim in the first round, then he would have to
form a pair with Ritesh or Suresh in the second round.
He would be free to pair with Qasim again in the third
round. In each round, each pair decided whether to play
the game in that round or not. If they decided not to
play, then no money was exchanged between them. If
they decided to play, they had to bet either Rs. 1 or
Rs. 2 in that round. For example, if they chose to bet
Rs. 2, then the player winning the game got Rs. 2 from
the one losing the game.
At the beginning of the tournament, the players had
Rs. 10 each. The following table shows partial information
about the amounts that the players had at the end of
each of the eight rounds. It shows every time a player
had Rs. 10 at the end of a round, as well as every time,
at the end of a round, a player had either the minimum
or the maximum amount that he would have had across
the eight rounds. For example, Suresh had Rs.10 at
the end of Rounds 1, 3, and 8 and not after any of the
other rounds. The maximum amount that he had at the
end of any round was Rs. 13 (at the end of Round 5),
and the minimum amount he had at the end of any round
was Rs. 8 (at the end of Round 2). At the end of all other
rounds, he must have had either Rs. 9, Rs. 11, or
Rs. 12.
It was also known that Pulak and Qasim had the same
amount of money with them at the end of Round 4.

Correct Answer
3
Explanation

Ritesh had exactly Rs.6 at the end of Round 8.
Correct Answer
4
Explanation

Pulak had exactly Rs.12 at the end of Round 6.
Correct Answer
6
Explanation

Ritesh had exactly Rs.6 at the end of Round 4.
Correct Answer
6
Explanation

From the table we can see that the amounts
increased or decreased by 2 at 12 instances, so
there are exactly 6 matches that were paid with a
bet of Rs.2.
Correct Answer
3
Explanation

In Round 5 Pulak and Ritesh played against each
other.
Directions for questions (11 to 15): There are only four
neighbourhoods in a city – Levmisto, Tyhrmisto,
Pesmisto and Kitmisto. During the onset of a pandemic,
the number of new cases of a disease in each of these
neighbourhoods was recorded over a period of five days.
On each day, the number of new cases recorded in any
of the neighbourhoods was either 0, 1, 2 or 3.
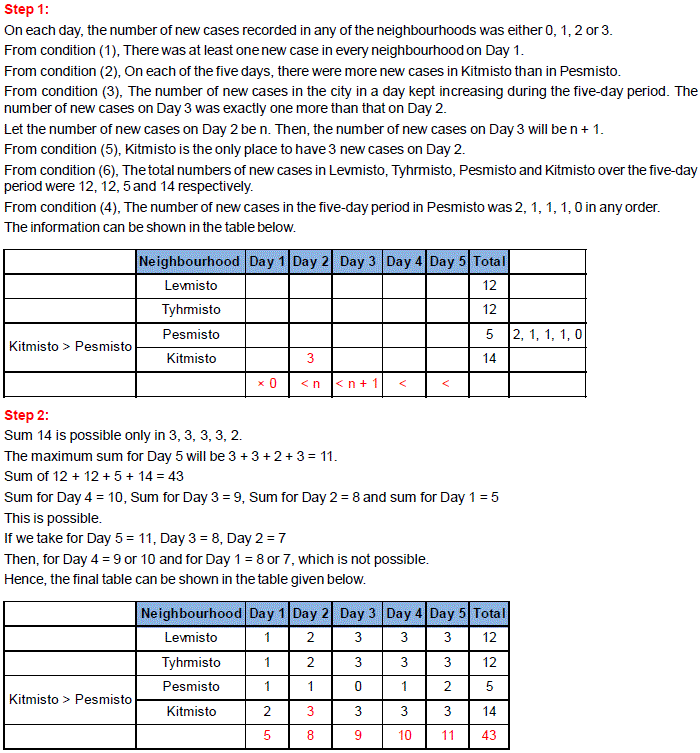
The following facts are also known:
1. There was at least one new case in every
neighbourhood on Day 1.
2. On each of the five days, there were more new cases
in Kitmisto than in Pesmisto.
3. The number of new cases in the city in a day kept
increasing during the five-day period.
The number of new cases on Day 3 was exactly one
more than that on Day 2.
4. The maximum number of new cases in a day in
Pesmisto was 2, and this happened only once during
the five-day period.
5. Kitmisto is the only place to have 3 new cases on
Day 2.
6. The total numbers of new cases in Levmisto,
Tyhrmisto, Pesmisto and Kitmisto over the five-day
period were 12, 12, 5 and 14 respectively.
Correct Answer
1
Explanation
The total number of new cases in the city on Day 2 was 8.
Correct Answer
4
Explanation

The number of new cases in Levmisto on Day 3 was 3.
Correct Answer
2
Explanation

Only on Day 3 Pesmisto did not have any new case.
Correct Answer
4
Explanation

Both Statement A and Statement B are necessarily false.
Correct Answer
4
Explanation

All 5 days Levmisto and Tyhrmisto have the same number of new cases.
Directions for questions (16 to 20): In the following, a year
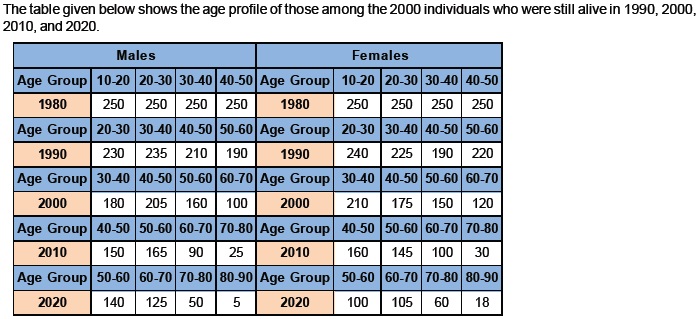
corresponds to 1st of January of that year.
A study to determine the mortality rate for a disease
began in 1980. The study chose 1000 males and 1000
females and followed them for forty years or until they
died, whichever came first. The 1000 males chosen in
1980 consisted of 250 each of ages 10 to less than 20,
20 to less than 30, 30 to less than 40, and 40 to less
than 50. The 1000 females chosen in 1980 also
consisted of 250 each of ages 10 to less than 20, 20 to
less than 30, 30 to less than 40, and 40 to less than 50.
The four figures below depict the age profile of those
among the 2000 individuals who were still alive in 1990,
2000, 2010, and 2020. The blue bars in each figure
represent the number of males in each age group at
that point in time, while the pink bars represent the
number of females in each age group at that point in
time. The numbers next to the bars give the exact
numbers being represented by the bars. For example, we know that 230 males among those tracked and who were
alive in 1990 were aged between 20 and 30.

Correct Answer
3
Explanation
In 2020, number of dead males
= 1000 – (180 + 205 + 160 + 100) = 355
In 2020, number of dead females
= 1000 – (210 + 175 + 150 + 120) = 345
Hence, required ratio = 355 : 345 = 71 : 69.
Correct Answer
4
Explanation

The number of people who were being tracked and
who were between 30 and 40 years of age in 1980
survived until 2010 = 90 + 100 = 190.
Correct Answer
4
Explanation

The number of individuals who were being tracked
and who were less than 30 years of age in 1980
survived until 2020 = 140 + 125 + 100 + 105 = 470.
Correct Answer
40
Explanation

Number of the males who were being tracked and
who were between 20 and 30 years of age in 1980
died in the period 2000 to 2010 = 205 – 165 = 40.
Correct Answer
30
Explanation

Number of the females who were being tracked
and who were between 20 and 30 years of age in
1980 died between the ages of 50 and 60
= 175 – 145 = 30.






















