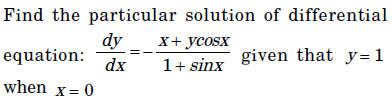Chapter 9: Differential Equations
Important Questions
1. Find the differential equation representing the curve y = cx + c2.
2. Write the differential equations representing the family of curves y = mx, where m is an arbitrary constant.
3. Find the differential equation representing the family of curves 5 = aebx+5, where a and b are arbitrary constants.
4. Find the differential equation for all the straight lines, which are at a unit distance from the origin.
5. Prove that x2 – y2 = c(x2 + y2)2 is the general solution of the differential equation
(x3 – 3xy2)dx = (y3 – 3x2y)dy, where C is a parameter
, given that y = 1
when x = 0
