Chapter 11: Dual Nature of Radiation and Matter
Important Questions
1. The given graph shows the variation of photoelectric current (I) versus applied voltage (V) for two different photosensitive materials and for two different intensities of the incident radiations. Identify the pairs of curves that correspond to different materials but same intensity of incident radiation.
2. Draw graphs showing variation of photoelectric current with applied voltage for two incident radiations of equal frequency and different intensities. Mark the graph for the radiation of higher intensity.
3. A 12.5 eV electron beam is used to excite a gaseous hydrogen atom at room temperature. Determine the wavelengths and the corresponding series of the lines emitted.
4. An electron and a photon each have a wavelength 1.00 nm. Find
(a) their momenta,
(b) the energy of the photon and
(c) the kinetic energy
5. (a) What is the significance of negative sign in the expression for the energy?
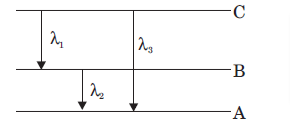
(b) Draw the energy level diagram showing how the line spectra corresponding to Paschen series occur due to transition between energy levels.